Πέμπτη 8 Δεκεμβρίου 2016
Παρασκευή 4 Νοεμβρίου 2016
TERENCE TAO's 246A, Notes 0: the complex numbers | What's new
246A, Notes 0: the complex numbers | What's new
Kronecker is famously reported to have said, “God created the natural
numbers; all else is the work of man”. The truth of this statement
(literal or otherwise) is debatable; but one can certainly view the
other standard number systems as (iterated) completions of the natural numbers
as (iterated) completions of the natural numbers 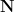 in various senses. For instance:
in various senses. For instance:
 from scratch as the metric completion of the rationals
from scratch as the metric completion of the rationals  , because the definition of a metric space itself requires the notion of the reals! (One can of course construct
, because the definition of a metric space itself requires the notion of the reals! (One can of course construct  by other means, for instance by using Dedekind cuts or by using uniform spaces
by other means, for instance by using Dedekind cuts or by using uniform spaces
in place of metric spaces.) The definition of the complex numbers as
the algebraic completion of the reals does not suffer from such a
non-circularity issue, but a certain amount of field theory is required
to work with this definition initially. For the purposes of quickly
constructing the complex numbers, it is thus more traditional to first
define as a quadratic extension of the reals
as a quadratic extension of the reals  , and more precisely as the extension
, and more precisely as the extension  formed by adjoining a square root
formed by adjoining a square root 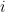 of
of 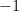 to the reals, that is to say a solution to the equation
to the reals, that is to say a solution to the equation  . It is not immediately obvious that this extension is in fact algebraically closed; this is the content of the famous fundamental theorem of algebra, which we will prove later in this course.
. It is not immediately obvious that this extension is in fact algebraically closed; this is the content of the famous fundamental theorem of algebra, which we will prove later in this course.
The two equivalent definitions of
– as the algebraic closure, and as a quadratic extension, of the reals
respectively – each reveal important features of the complex numbers in
applications. Because
is algebraically closed, all polynomials over the complex numbers split
completely, which leads to a good spectral theory for both
finite-dimensional matrices and infinite-dimensional operators; in
particular, one expects to be able to diagonalise most matrices and
operators. Applying this theory to constant coefficient ordinary
differential equations leads to a unified theory of such solutions, in
which real-variable ODE behaviour such as exponential growth or decay,
polynomial growth, and sinusoidal oscillation all become aspects of a
single object, the complex exponential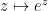 (or more generally, the matrix exponential
(or more generally, the matrix exponential  ).
).
Applying this theory more generally to diagonalise arbitrary
translation-invariant operators over some locally compact abelian group,
one arrives at Fourier analysis,
which is thus most naturally phrased in terms of complex-valued
functions rather than real-valued ones. If one drops the assumption that
the underlying group is abelian, one instead discovers the
representation theory of unitary representations,
which is simpler to study than the real-valued counterpart of
orthogonal representations. For closely related reasons, the theory of
complex Lie groups is simpler than that of real Lie groups.
Meanwhile, the fact that the complex numbers are a quadratic extension
of the reals lets one view the complex numbers geometrically as a
two-dimensional plane over the reals (the Argand plane).
Whereas a point singularity in the real line disconnects that line, a
point singularity in the Argand plane leaves the rest of the plane
connected (although, importantly, the punctured plane is no longer simply connected).
As we shall see, this fact causes singularities in complex analytic
functions to be better behaved than singularities of real analytic
functions, ultimately leading to the powerful residue calculus
for computing complex integrals. Remarkably, this calculus, when
combined with the quintessentially complex-variable technique of contour shifting, can also be used to compute some (though certainly not all) definite integrals of real-valued
functions that would be much more difficult to compute by purely
real-variable methods; this is a prime example of Hadamard’s famous
dictum that “the shortest path between two truths in the real domain
passes through the complex domain”.
Another important geometric feature of the Argand plane is the angle
between two tangent vectors to a point in the plane. As it turns out,
the operation of multiplication by a complex scalar preserves the
magnitude and orientation of such angles; the same fact is true for any
non-degenerate complex analytic mapping, as can be seen by performing a
Taylor expansion to first order. This fact ties the study of complex
mappings closely to that of the conformal geometry
of the plane (and more generally, of two-dimensional surfaces and
domains). In particular, one can use complex analytic maps to
conformally transform one two-dimensional domain to another, leading
among other things to the famous Riemann mapping theorem, and to the classification of Riemann surfaces.
If one Taylor expands complex analytic maps to second order rather than
first order, one discovers a further important property of these maps,
namely that they are harmonic.
This fact makes the class of complex analytic maps extremely rigid and
well behaved analytically; indeed, the entire theory of elliptic PDE now
comes into play, giving useful properties such as elliptic regularity and the maximum principle.
In fact, due to the magic of residue calculus and contour shifting, we
already obtain these properties for maps that are merely complex
differentiable rather than complex analytic, which leads to the striking
fact that complex differentiable functions are automatically analytic
(in contrast to the real-variable case, in which real differentiable
functions can be very far from being analytic).
The geometric structure of the complex numbers (and more generally of
complex manifolds and complex varieties), when combined with the
algebraic closure of the complex numbers, leads to the beautiful subject
of complex algebraic geometry, which motivates the much more
general theory developed in modern algebraic geometry. However, we will
not develop the algebraic geometry aspects of complex analysis here.
Last, but not least, because of the good behaviour of Taylor series in
the complex plane, complex analysis is an excellent setting in which to
manipulate various generating functions, particularly Fourier series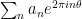 (which can be viewed as boundary values of power (or Laurent) series
(which can be viewed as boundary values of power (or Laurent) series  ), as well as Dirichlet series
), as well as Dirichlet series  . The theory of contour integration provides a very useful dictionary between the asymptotic behaviour of the sequence
. The theory of contour integration provides a very useful dictionary between the asymptotic behaviour of the sequence  ,
,
and the complex analytic behaviour of the Dirichlet or Fourier series,
particularly with regard to its poles and other singularities. This
turns out to be a particularly handy dictionary in analytic number theory, for instance relating the distribution of the primes to the Riemann zeta function. Nowadays, many of the analytic number theory results first obtained through complex analysis (such as the prime number theorem)
can also be obtained by more “real-variable” methods; however the
complex-analytic viewpoint is still extremely valuable and illuminating.
We will frequently touch upon many of these connections to other fields
of mathematics in these lecture notes. However, these are mostly side
remarks intended to provide context, and it is certainly possible to
skip most of these tangents and focus purely on the complex analysis
material in these notes if desired.
Note: complex analysis is a very visual subject, and one should draw
plenty of pictures while learning it. I am however not planning to put
too many pictures in these notes, partly as it is somewhat inconvenient
to do so on this blog from a technical perspective, but also because
pictures that one draws on one’s own are likely to be far more useful to
you than pictures that were supplied by someone else.
the course; we are concentrating almost all of the algebraic
preliminaries in this section in order to get them out of the way and
focus subsequently on the analytic aspects of the complex numbers.
Thanks to the laws of high-school algebra, we know that the real numbers are a field:
are a field:
it is endowed with the arithmetic operations of addition, subtraction,
multiplication, and division, as well as the additive identity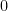 and multiplicative identity
and multiplicative identity 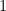 , that obey the usual laws of algebra (i.e. the field axioms).
, that obey the usual laws of algebra (i.e. the field axioms).
The algebraic structure of the reals does have one drawback though – not
all (non-trivial) polynomials have roots! Most famously, the polynomial
equation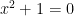 has no solutions over the reals, because
has no solutions over the reals, because  is always non-negative, and hence
is always non-negative, and hence  is always strictly positive, whenever
is always strictly positive, whenever  is a real number.
is a real number.
As mentioned in the introduction, one traditional way to define the complex numbers is as the smallest possible extension of the reals
is as the smallest possible extension of the reals  that fixes this one specific problem:
that fixes this one specific problem:

as a given in this course; constructions of the real number system can
of course be found in many real analysis texts, including my own.)
Definition 1 is short, but proposing it as a definition of the complex numbers raises some immediate questions:
We begin with existence. One can construct the complex numbers quite
explicitly and quickly using the Argand plane construction; see Remark 7
below. However, from the perspective of higher mathematics, it is more
natural to view the construction of the complex numbers as a special
case of the more general algebraic construction that can extend any
field by the root
by the root 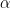 of an irreducible nonlinear polynomial
of an irreducible nonlinear polynomial ![{P \in k[\mathrm{x}]} {P \in k[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7BP+%5Cin+k%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) over that field; this produces a field of complex numbers
over that field; this produces a field of complex numbers  when specialising to the case where
when specialising to the case where  and
and 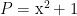 . We will just describe this construction in that special case, leaving the general case as an exercise.
. We will just describe this construction in that special case, leaving the general case as an exercise.
Starting with the real numbers , we can form the space
, we can form the space ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) of (formal) polynomials
of (formal) polynomials
 with real co-efficients
with real co-efficients  and arbitrary non-negative integer
and arbitrary non-negative integer  in one indeterminate variable
in one indeterminate variable  . (A small technical point: we do not view this indeterminate
. (A small technical point: we do not view this indeterminate  as belonging to any particular domain such as
as belonging to any particular domain such as  , so we do not view these polynomials
, so we do not view these polynomials 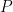 as functions but merely as formal expressions involving a placeholder symbol
as functions but merely as formal expressions involving a placeholder symbol  (which we have rendered in Roman type to indicate its formal character). In this particular characteristic zero setting of working over the reals, it turns out to be harmless to identify each polynomial
(which we have rendered in Roman type to indicate its formal character). In this particular characteristic zero setting of working over the reals, it turns out to be harmless to identify each polynomial 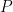 with the corresponding function
with the corresponding function 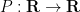 formed by interpreting the indeterminate
formed by interpreting the indeterminate 
as a real variable; but if one were to generalise this construction to
positive characteristic fields, and particularly finite fields, then one
can run into difficulties if polynomials are not treated formally, due
to the fact that two distinct formal polynomials might agree on all
inputs in a given finite field (e.g. the polynomials and
and  agree for all
agree for all  in the finite field
in the finite field  ). However, this subtlety can be ignored for the purposes of this course.) This space
). However, this subtlety can be ignored for the purposes of this course.) This space ![{{\bf R}[x]} {{\bf R}[x]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5Bx%5D%7D&bg=ffffff&fg=000000&s=0)
of polynomials has a pretty good algebraic structure, in particular the
usual operations of addition, subtraction, and multiplication on
polynomials, together with the zero polynomial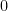 and the unit polynomial
and the unit polynomial 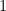 , give
, give ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) the structure of a (unital) commutative ring. This commutative ring also contains
the structure of a (unital) commutative ring. This commutative ring also contains  as a subring (identifying each real number
as a subring (identifying each real number  with the degree zero polynomial
with the degree zero polynomial  ). The ring
). The ring ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) is however not a field, because many non-zero elements of
is however not a field, because many non-zero elements of ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) do not have multiplicative inverses. (In fact, no non-constant polynomial in
do not have multiplicative inverses. (In fact, no non-constant polynomial in ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) has an inverse in
has an inverse in ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) , because the product of two non-constant polynomials has a degree that is the sum of the degrees of the factors.)
, because the product of two non-constant polynomials has a degree that is the sum of the degrees of the factors.)
If a unital commutative ring fails to be field, then it will instead possess a number of non-trivial ideals. The only ideal we will need to consider here is the principal ideal
![\displaystyle \langle \mathrm{x}^2+1 \rangle := \{ (\mathrm{x}^2+1) P(\mathrm{x}): P(\mathrm{x}) \in {\bf R}[\mathrm{x}] \}. \displaystyle \langle \mathrm{x}^2+1 \rangle := \{ (\mathrm{x}^2+1) P(\mathrm{x}): P(\mathrm{x}) \in {\bf R}[\mathrm{x}] \}.](https://s0.wp.com/latex.php?latex=%5Cdisplaystyle++%5Clangle+%5Cmathrm%7Bx%7D%5E2%2B1+%5Crangle+%3A%3D+%5C%7B+%28%5Cmathrm%7Bx%7D%5E2%2B1%29+P%28%5Cmathrm%7Bx%7D%29%3A+P%28%5Cmathrm%7Bx%7D%29+%5Cin+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D+%5C%7D.&bg=ffffff&fg=000000&s=0) This is clearly an ideal of
This is clearly an ideal of ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) – it is closed under addition and subtraction, and the product of any element of the ideal
– it is closed under addition and subtraction, and the product of any element of the ideal 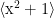 with an element of the full ring
with an element of the full ring ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) remains in the ideal
remains in the ideal 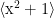 .
.
We now define to be the quotient space
to be the quotient space
![\displaystyle {\bf C} := {\bf R}[\mathrm{x}] / \langle \mathrm{x}^2+1 \rangle \displaystyle {\bf C} := {\bf R}[\mathrm{x}] / \langle \mathrm{x}^2+1 \rangle](https://s0.wp.com/latex.php?latex=%5Cdisplaystyle+%7B%5Cbf+C%7D+%3A%3D+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D+%2F+%5Clangle+%5Cmathrm%7Bx%7D%5E2%2B1+%5Crangle&bg=ffffff&fg=000000&s=0) of the commutative ring
of the commutative ring ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) by the ideal
by the ideal 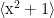 ; this is the space of cosets
; this is the space of cosets  of
of 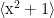 in
in ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) . Because
. Because 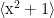 is an ideal, there is an obvious way to define addition, subtraction, and multiplication in
is an ideal, there is an obvious way to define addition, subtraction, and multiplication in  , namely by setting
, namely by setting

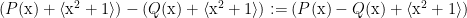 and
and
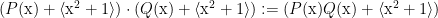 for all
for all ![{P(\mathrm{x}), Q(\mathrm{x}) \in {\bf R}[\mathrm{x}]} {P(\mathrm{x}), Q(\mathrm{x}) \in {\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7BP%28%5Cmathrm%7Bx%7D%29%2C+Q%28%5Cmathrm%7Bx%7D%29+%5Cin+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) ; these operations, together with the additive identity
; these operations, together with the additive identity 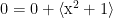 and the multiplicative identity
and the multiplicative identity  , can be easily verified to give
, can be easily verified to give  the structure of a commutative ring. Also, the real line
the structure of a commutative ring. Also, the real line  embeds into
embeds into  by identifying each real number
by identifying each real number  with the coset
with the coset 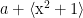 ; note that this identification is injective, as no real number is a multiple of the polynomial
; note that this identification is injective, as no real number is a multiple of the polynomial 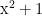 .
.
If we define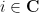 to be the coset
to be the coset
 then it is clear from construction that
then it is clear from construction that  . Thus
. Thus  contains both
contains both  and a solution of the equation
and a solution of the equation  . Also, since every element of
. Also, since every element of  is of the form
is of the form 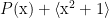 for some polynomial
for some polynomial ![{P \in {\bf R}[\mathrm{x}]} {P \in {\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7BP+%5Cin+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) , we see that every element of
, we see that every element of  is a polynomial combination
is a polynomial combination  of
of 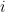 with real coefficients; in particular, any subring of
with real coefficients; in particular, any subring of  that contains
that contains  and
and 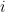 will necessarily have to contain every element of
will necessarily have to contain every element of  . Thus
. Thus  is generated by
is generated by  and
and 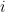 .
.
The only remaining thing to verify is that is a field and not just a commutative ring. In other words, we need to show that every non-zero element of
is a field and not just a commutative ring. In other words, we need to show that every non-zero element of  has a multiplicative inverse. This stems from a particular property of the polynomial
has a multiplicative inverse. This stems from a particular property of the polynomial 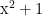 , namely that it is irreducible in
, namely that it is irreducible in ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) . That is to say, we cannot factor
. That is to say, we cannot factor 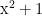 into non-constant polynomials
into non-constant polynomials
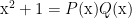 with
with ![{P(\mathrm{x}), Q(\mathrm{x}) \in {\bf R}[\mathrm{x}]} {P(\mathrm{x}), Q(\mathrm{x}) \in {\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7BP%28%5Cmathrm%7Bx%7D%29%2C+Q%28%5Cmathrm%7Bx%7D%29+%5Cin+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) . Indeed, as
. Indeed, as 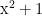 has degree two, the only possible way such a factorisation could occur is if
has degree two, the only possible way such a factorisation could occur is if  both have degree one, which would imply that the polynomial
both have degree one, which would imply that the polynomial  has a root in the reals
has a root in the reals  , which of course it does not.
, which of course it does not.
Because the polynomial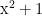 is irreducible, it is also prime: if
is irreducible, it is also prime: if 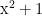 divides a product
divides a product  of two polynomials in
of two polynomials in ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) , then it must also divide at least one of the factors
, then it must also divide at least one of the factors 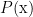 ,
,  . Indeed, if
. Indeed, if 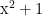 does not divide
does not divide 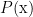 , then by irreducibility the greatest common divisor of
, then by irreducibility the greatest common divisor of 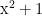 and
and 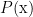 is
is 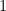 . Applying the Euclidean algorithm for polynomials, we then obtain a representation of
. Applying the Euclidean algorithm for polynomials, we then obtain a representation of 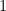 as
as
 for some polynomials
for some polynomials 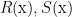 ; multiplying both sides by
; multiplying both sides by  , we conclude that
, we conclude that  is a multiple of
is a multiple of 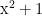 .
.
Since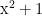 is prime, the quotient space
is prime, the quotient space ![{{\bf C} = {\bf R}[\mathrm{x}] / \langle \mathrm{x}^2+1 \rangle} {{\bf C} = {\bf R}[\mathrm{x}] / \langle \mathrm{x}^2+1 \rangle}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+C%7D+%3D+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D+%2F+%5Clangle+%5Cmathrm%7Bx%7D%5E2%2B1+%5Crangle%7D&bg=ffffff&fg=000000&s=0) is an integral domain: there are no zero-divisors in
is an integral domain: there are no zero-divisors in  other than zero. This brings us closer to the task of showing that
other than zero. This brings us closer to the task of showing that  is a field, but we are not quite there yet; note for instance that
is a field, but we are not quite there yet; note for instance that ![{{\bf R}[\mathrm{x}]} {{\bf R}[\mathrm{x}]}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D%7D&bg=ffffff&fg=000000&s=0) is an integral domain, but not a field. But one can finish up by using finite dimensionality. As
is an integral domain, but not a field. But one can finish up by using finite dimensionality. As  is a ring containing the field
is a ring containing the field  , it is certainly a vector space over
, it is certainly a vector space over  ; as
; as  is generated by
is generated by  and
and 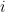 , and
, and 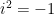 , we see that it is in fact a two-dimensional vector space over
, we see that it is in fact a two-dimensional vector space over  , spanned by
, spanned by 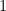 and
and 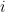 (which are linearly independent, as
(which are linearly independent, as 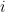 clearly cannot be real). In particular, it is finite dimensional. For any non-zero
clearly cannot be real). In particular, it is finite dimensional. For any non-zero  , the multiplication map
, the multiplication map  is an
is an  -linear map from this finite-dimensional vector space to itself. As
-linear map from this finite-dimensional vector space to itself. As  is an integral domain, this map is injective; by finite-dimensionality, it is therefore surjective (by the rank-nullity theorem). In particular, there exists
is an integral domain, this map is injective; by finite-dimensionality, it is therefore surjective (by the rank-nullity theorem). In particular, there exists  such that
such that  , and hence
, and hence  is invertible and
is invertible and  is a field. This concludes the construction of a complex field
is a field. This concludes the construction of a complex field  .
.
 up to isomorphism is a straightforward exercise:
up to isomorphism is a straightforward exercise:
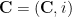 as the
as the
complex field; the other complex fields out there will no longer be of
much importance in this course (or indeed, in most of mathematics), with
one small exception that we will get to later in this section. One can,
if one wishes, use the above abstract algebraic construction![{( {\bf R}[\mathrm{x}] / \langle \mathrm{x}^2+1 \rangle, \mathrm{x} + \langle \mathrm{x}^2 + 1 \rangle)} {( {\bf R}[\mathrm{x}] / \langle \mathrm{x}^2+1 \rangle, \mathrm{x} + \langle \mathrm{x}^2 + 1 \rangle)}](https://s0.wp.com/latex.php?latex=%7B%28+%7B%5Cbf+R%7D%5B%5Cmathrm%7Bx%7D%5D+%2F+%5Clangle+%5Cmathrm%7Bx%7D%5E2%2B1+%5Crangle%2C+%5Cmathrm%7Bx%7D+%2B+%5Clangle+%5Cmathrm%7Bx%7D%5E2+%2B+1+%5Crangle%29%7D&bg=ffffff&fg=000000&s=0) as the choice for “the” complex field
as the choice for “the” complex field  , but one can certainly pick other choices if desired (e.g. the Argand plane construction in Remark 7 below). But in view of Exercise 4, the precise construction of
, but one can certainly pick other choices if desired (e.g. the Argand plane construction in Remark 7 below). But in view of Exercise 4, the precise construction of 
is not terribly relevant for the purposes of actually doing complex
analysis, much as the question of whether to construct the real numbers
using Dedekind cuts, equivalence classes of Cauchy sequences, or some
other construction is not terribly relevant for the purposes of actually
doing real analysis. So, from here on out, we will no longer refer to
the precise construction of used; the reader may certainly substitute his or her own favourite construction of
used; the reader may certainly substitute his or her own favourite construction of  in place of
in place of ![{{\bf R}[\mathbf{x}] / \langle {\mathbf x}^2 + 1 \rangle} {{\bf R}[\mathbf{x}] / \langle {\mathbf x}^2 + 1 \rangle}](https://s0.wp.com/latex.php?latex=%7B%7B%5Cbf+R%7D%5B%5Cmathbf%7Bx%7D%5D+%2F+%5Clangle+%7B%5Cmathbf+x%7D%5E2+%2B+1+%5Crangle%7D&bg=ffffff&fg=000000&s=0) if desired, with essentially no change to the rest of the lecture notes.
if desired, with essentially no change to the rest of the lecture notes.
 of the form
of the form  for
for 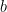 real are known as purely imaginary numbers;
real are known as purely imaginary numbers;
the terminology is colourful, but despite the name, imaginary numbers
have precisely the same first-class mathematical object status as real
numbers. If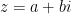 is a complex number, the real components
is a complex number, the real components  of
of  are known as the real part
are known as the real part 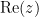 and imaginary part
and imaginary part 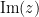 of
of  respectively. Complex numbers that are not real are occasionally referred to as strictly complex numbers. In the complex plane, the set
respectively. Complex numbers that are not real are occasionally referred to as strictly complex numbers. In the complex plane, the set  of real numbers forms the real axis, and the set
of real numbers forms the real axis, and the set  of imaginary numbers forms the imaginary axis. Traditionally, elements of
of imaginary numbers forms the imaginary axis. Traditionally, elements of  are denoted with symbols such as
are denoted with symbols such as  ,
,  , or
, or 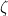 , while symbols such as
, while symbols such as 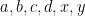 are typically intended to represent real numbers instead.
are typically intended to represent real numbers instead.
 to a non-negative integer
to a non-negative integer 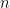 by declaring inductively
by declaring inductively 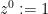 and
and  for
for 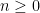 ; in particular we adopt the usual convention that
; in particular we adopt the usual convention that  (when thinking of the base
(when thinking of the base 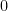 as a complex number, and the exponent
as a complex number, and the exponent 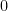 as a non-negative integer). For negative integers
as a non-negative integer). For negative integers 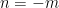 , we define
, we define  for non-zero
for non-zero  ; we leave
; we leave  undefined when
undefined when  is zero and
is zero and 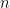 is negative. At the present time we do not attempt to define
is negative. At the present time we do not attempt to define 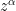 for any exponent
for any exponent 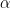
other than an integer; we will return to such exponentiation operations
later in the course, though we will at least define the complex
exponential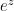 for any complex
for any complex  later in this set of notes.
later in this set of notes.
By definition, a complex field is a field
is a field  together with a root
together with a root  of the equation
of the equation 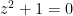 . But if
. But if  is a root of the equation
is a root of the equation 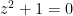 , then so is
, then so is 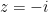 (indeed, from the factorisation
(indeed, from the factorisation  we see that these are the only two roots of this quadratic equation. Thus we have another complex field
we see that these are the only two roots of this quadratic equation. Thus we have another complex field  which differs from
which differs from  only in the choice of root
only in the choice of root 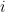 . By Exercise 4, there is a unique field isomorphism from
. By Exercise 4, there is a unique field isomorphism from  to
to  that maps
that maps 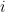 to
to 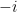 (i.e. a complex field isomorphism from
(i.e. a complex field isomorphism from  to
to  ); this operation is known as complex conjugation and is denoted
); this operation is known as complex conjugation and is denoted  . In coordinates, we have
. In coordinates, we have
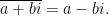 Being a field isomorphism, we have in particular that
Being a field isomorphism, we have in particular that
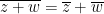 and
and
 for all complex numbers
for all complex numbers  . It is also clear that complex conjugation fixes the real numbers, and only the real numbers:
. It is also clear that complex conjugation fixes the real numbers, and only the real numbers: 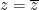 if and only if
if and only if 
is real. Geometrically, complex conjugation is the operation of
reflection in the complex plane across the real axis. It is clearly an involution in the sense that it is its own inverse:
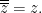 One can also relate the real and imaginary parts to complex conjugation via the identities
One can also relate the real and imaginary parts to complex conjugation via the identities
 by its complex conjugate
by its complex conjugate 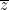 , we obtain a quantity
, we obtain a quantity  which is invariant with respect to conjugation (i.e.
which is invariant with respect to conjugation (i.e.  ) and is therefore real. The map
) and is therefore real. The map  produced this way is known in field theory as the norm form of
produced this way is known in field theory as the norm form of  over
over  ; it is clearly multiplicative in the sense that
; it is clearly multiplicative in the sense that 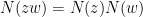 , and is only zero when
, and is only zero when  is zero. It can be used to link multiplicative inversion with complex conjugation, in that we clearly have
is zero. It can be used to link multiplicative inversion with complex conjugation, in that we clearly have
for any non-zero complex number . In coordinates, we have
. In coordinates, we have
 (thus recovering, by the way, the inversion formula
(thus recovering, by the way, the inversion formula 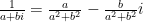 implicit in Remark 7). In coordinates, the multiplicativity
implicit in Remark 7). In coordinates, the multiplicativity 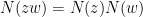 takes the form of Lagrange’s identity
takes the form of Lagrange’s identity
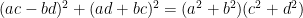
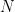 of the complex numbers has the feature of being positive definite:
of the complex numbers has the feature of being positive definite: 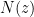 is always non-negative (and strictly positive when
is always non-negative (and strictly positive when  is non-zero). This is a feature that is somewhat special to the complex numbers; for instance, the quadratic extension
is non-zero). This is a feature that is somewhat special to the complex numbers; for instance, the quadratic extension 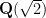 of the rationals
of the rationals  by
by 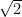 has the norm form
has the norm form 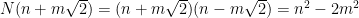 , which is indefinite. One can view this positive definiteness of the norm form as the one remaining vestige in
, which is indefinite. One can view this positive definiteness of the norm form as the one remaining vestige in  of the order structure
of the order structure 
on the reals, which as remarked previously is no longer present
directly in the complex numbers. (One can also view the positive
definiteness of the norm form as a consequence of the topological
connectedness of the punctured complex plane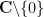 : the norm form is positive at
: the norm form is positive at 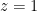 , and cannot change sign anywhere in
, and cannot change sign anywhere in 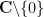 , so is forced to be positive on the rest of this connected region.)
, so is forced to be positive on the rest of this connected region.)
One consequence of positive definiteness is that the bilinear form
 becomes a positive definite inner product on
becomes a positive definite inner product on  (viewed as a vector space over
(viewed as a vector space over  ). In particular, this turns the complex numbers into an inner product space over the reals. From the usual theory of inner product spaces, we can then construct a norm
). In particular, this turns the complex numbers into an inner product space over the reals. From the usual theory of inner product spaces, we can then construct a norm
 (thus, the norm is the square root of the norm form) which obeys the triangle inequality
(thus, the norm is the square root of the norm form) which obeys the triangle inequality
(which implies the usual permutations of this inequality, such as ), and from the multiplicativity of the norm form we also have
), and from the multiplicativity of the norm form we also have
(and hence also if
if  is non-zero) and from the involutive nature of complex conjugation we have
is non-zero) and from the involutive nature of complex conjugation we have
The norm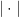 clearly extends the absolute value operation
clearly extends the absolute value operation 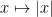 on the real numbers, and so we also refer to the norm
on the real numbers, and so we also refer to the norm 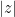 of a complex number
of a complex number  as its absolute value or magnitude. In coordinates, we have
as its absolute value or magnitude. In coordinates, we have
thus for instance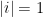 , and from (6) we also immediately have the useful inequalities
, and from (6) we also immediately have the useful inequalities
As with any other normed vector space, the norm defines a metric on the complex numbers via the definition
defines a metric on the complex numbers via the definition
 Note that using the Argand plane representation of
Note that using the Argand plane representation of  as
as 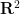 that this metric coincides with the usual Euclidean metric on
that this metric coincides with the usual Euclidean metric on 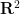 . This metric in turn defines a topology on
. This metric in turn defines a topology on  (generated in the usual manner by the open disks
(generated in the usual manner by the open disks 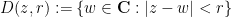 ),
),
which in turn generates all the usual topological notions such as the
concept of an open set, closed set, compact set, connected set, and
boundary of a set; the notion of a limit of a sequence ; the notion of a continuous map, and so forth. For instance, a sequence
; the notion of a continuous map, and so forth. For instance, a sequence  of complex numbers converges to a limit
of complex numbers converges to a limit  if
if 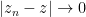 as
as 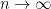 , and a map
, and a map  is continuous if one has
is continuous if one has  whenever
whenever  ,
,
or equivalently if the inverse image of any open set is open. Again,
using the Argand plane representation, these notions coincide with their
counterparts on the Euclidean plane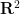 .
.
As usual, if a sequence of complex numbers converges to a limit
of complex numbers converges to a limit  , we write
, we write 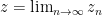 . From the triangle inequality (3) and the multiplicativity (4) we see that the addition operation
. From the triangle inequality (3) and the multiplicativity (4) we see that the addition operation  , subtraction operation
, subtraction operation  , and multiplication operation
, and multiplication operation 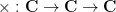 , thus we have the familiar limit laws
, thus we have the familiar limit laws

 and
and
 whenever the limits on the right-hand side exist. Similarly, from (5) we see that complex conjugation is an isometry of the complex numbers, thus
whenever the limits on the right-hand side exist. Similarly, from (5) we see that complex conjugation is an isometry of the complex numbers, thus
 when the limit on the right-hand side exists. As a consequence, the norm form
when the limit on the right-hand side exists. As a consequence, the norm form  and the absolute value
and the absolute value  are also continuous, thus
are also continuous, thus
 whenever the limit on the right-hand side exists. Using the formula (2)
whenever the limit on the right-hand side exists. Using the formula (2)
for the reciprocal of a complex number, we also see that division is a
continuous operation as long as the denominator is non-zero, thus
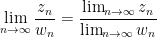 as long as the limits on the right-hand side exist, and the limit in the denominator is non-zero.
as long as the limits on the right-hand side exist, and the limit in the denominator is non-zero.
From (7) we see that
 in particular
in particular
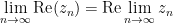 and
and
 whenever the limit on the right-hand side exists. One consequence of this is that
whenever the limit on the right-hand side exists. One consequence of this is that  is complete: every sequence
is complete: every sequence  of complex numbers that is a Cauchy sequence (thus
of complex numbers that is a Cauchy sequence (thus  as
as 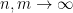 ) converges to a unique complex limit
) converges to a unique complex limit  . (As such, one can view the complex numbers as a (very small) example of a Hilbert space.)
. (As such, one can view the complex numbers as a (very small) example of a Hilbert space.)
As with the reals, we have the fundamental fact that any formal series of complex numbers which is absolutely convergent, in the sense that the non-negative series
of complex numbers which is absolutely convergent, in the sense that the non-negative series  is finite, is necessarily convergent to some complex number
is finite, is necessarily convergent to some complex number  , in the sense that the partial sums
, in the sense that the partial sums  converge to
converge to  as
as 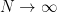 . This is because the triangle inequality ensures that the partial sums are a Cauchy sequence. As usual we write
. This is because the triangle inequality ensures that the partial sums are a Cauchy sequence. As usual we write  to denote the assertion that
to denote the assertion that  is the limit of the partial sums
is the limit of the partial sums  .
.
We will occasionally have need to deal with series that are only
conditionally convergent rather than absolutely convergent, but in most
of our applications the only series we will actually evaluate are the
absolutely convergent ones. Many of the limit laws imply analogues for
series, thus for instance
 whenever the series on the right-hand side is absolutely convergent
whenever the series on the right-hand side is absolutely convergent
(or even just convergent). We will not write down an exhaustive list of
such series laws here.
An important role in complex analysis is played by the unit circle
 In coordinates, this is the set of points
In coordinates, this is the set of points 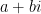 for which
for which 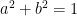 ,
,
and so this indeed has the geometric structure of a unit circle.
Elements of the unit circle will be referred to in these notes as phases. Every non-zero complex number has a unique polar decomposition as
has a unique polar decomposition as 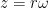 where
where 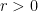 is a positive real and
is a positive real and 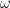 lies on the unit circle
lies on the unit circle  . Indeed, it is easy to see that this decomposition is given by
. Indeed, it is easy to see that this decomposition is given by 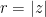 and
and 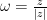 , and that this is the only polar decopmosition of
, and that this is the only polar decopmosition of  . We refer to the polar components
. We refer to the polar components  and
and  of a non-zero complex number
of a non-zero complex number  as the magnitude and phase of
as the magnitude and phase of  respectively.
respectively.
From (4) we see that the unit circle is a multiplicative group; it contains the multiplicative identity
is a multiplicative group; it contains the multiplicative identity 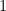 , and if
, and if  lie in
lie in  , then so do
, then so do  and
and  . From (2) we see that reciprocation and complex conjugation agree on the unit circle, thus
. From (2) we see that reciprocation and complex conjugation agree on the unit circle, thus
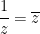 for
for  . It is worth emphasising that this useful identity does not hold as soon as one leaves the unit circle, in which case one must use the more general formula (2) instead! If
. It is worth emphasising that this useful identity does not hold as soon as one leaves the unit circle, in which case one must use the more general formula (2) instead! If  are non-zero complex numbers
are non-zero complex numbers  with polar decompositions
with polar decompositions 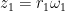 and
and  respectively, then clearly the polar decompositions of
respectively, then clearly the polar decompositions of 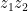 and
and  are given by
are given by  and
and 
respectively. Thus polar coordinates are very convenient for performing
complex multiplication, although they turn out to be atrocious for
performing complex addition. (This can be contrasted with the usual
Cartesian coordinates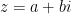 ,
,
which are very convenient for performing complex addition and mildly
inconvenient for performing complex multiplication.) In the language of
group theory, the polar decomposition splits the multiplicative complex
group as the direct product of the positive reals
as the direct product of the positive reals 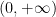 and the unit circle
and the unit circle  :
:  .
.
If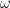 is an element of the unit circle
is an element of the unit circle  , then from (4) we see that the operation
, then from (4) we see that the operation 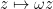 of multiplication by
of multiplication by 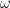 is an isometry of
is an isometry of  , in the sense that
, in the sense that
 for all complex numbers
for all complex numbers  . This isometry also preserves the origin
. This isometry also preserves the origin 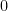 . As such, it is geometrically obvious (see Exercise 11 below) that the map
. As such, it is geometrically obvious (see Exercise 11 below) that the map 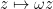
must either be a rotation around the origin, or a reflection around a
line. The former operation is orientation preserving, and the latter is
orientation reversing. Since the map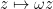 is clearly orientation preserving when
is clearly orientation preserving when 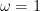 , and the unit circle
, and the unit circle  is connected, a continuity argument shows that
is connected, a continuity argument shows that 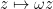 must be orientation preserving for all
must be orientation preserving for all 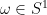 , and so must be a rotation around the origin by some angle. Of course, by trigonometry, we may write
, and so must be a rotation around the origin by some angle. Of course, by trigonometry, we may write
 for some real number
for some real number  . The rotation
. The rotation 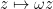 clearly maps the number
clearly maps the number 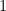 to the number
to the number 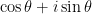 , and so the rotation must be a counter-clockwise rotation by
, and so the rotation must be a counter-clockwise rotation by  (adopting the usual convention of placing
(adopting the usual convention of placing 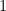 to the right of the origin and
to the right of the origin and 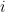 above it). In particular, when applying this rotation
above it). In particular, when applying this rotation 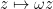 to another point
to another point 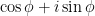 on the unit circle, this point must get rotated to
on the unit circle, this point must get rotated to  . We have thus given a geometric proof of the multiplication formula
. We have thus given a geometric proof of the multiplication formula
taking real and imaginary parts, we recover the familiar trigonometric addition formulae

 We can also iterate the multiplication formula to give de Moivre’s formula
We can also iterate the multiplication formula to give de Moivre’s formula
 for any natural number
for any natural number 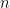 (or indeed for any integer
(or indeed for any integer 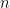 ), which can in turn be used to recover familiar identities such as the double angle formulae
), which can in turn be used to recover familiar identities such as the double angle formulae
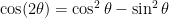
 or triple angle formulae
or triple angle formulae

 after expanding out de Moivre’s formula for
after expanding out de Moivre’s formula for 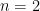 or
or  and taking real and imaginary parts.
and taking real and imaginary parts.
 can now be written in polar form as
can now be written in polar form as
with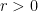 and
and 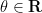 ; we refer to
; we refer to  as an argument of
as an argument of  ,
,
and can be interpreted as an angle of counterclockwise rotation needed
to rotate the positive real axis to a position that contains . The argument is not quite unique, due to the periodicity of sine and cosine: if
. The argument is not quite unique, due to the periodicity of sine and cosine: if  is an argument of
is an argument of  , then so is
, then so is 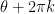 for any integer
for any integer  , and conversely these are all the possible arguments that
, and conversely these are all the possible arguments that  can have. The set of all such arguments will be denoted
can have. The set of all such arguments will be denoted  ; it is a coset of the discrete group
; it is a coset of the discrete group  , and can thus be viewed as an element of the
, and can thus be viewed as an element of the 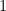 -torus
-torus 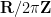 .
.
The operation of multiplying a complex number
of multiplying a complex number  by a given non-zero complex number
by a given non-zero complex number  now has a very appealing geometric interpretation when expressing
now has a very appealing geometric interpretation when expressing  in polar coordinates (9): this operation is the composition of the operation of dilation by
in polar coordinates (9): this operation is the composition of the operation of dilation by  around the origin, and counterclockwise rotation by
around the origin, and counterclockwise rotation by  around the origin. For instance, multiplication by
around the origin. For instance, multiplication by 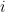 performs a counter-clockwise rotation by
performs a counter-clockwise rotation by 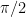 around the origin, while multiplication by
around the origin, while multiplication by 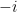 performs instead a clockwise rotation by
performs instead a clockwise rotation by 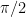 .
.
As complex multiplication is commutative and associative, it does not
matter in which order one performs the dilation and rotation operations.
Similarly, using Cartesian coordinates, we see that the operation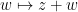 of adding a complex number
of adding a complex number  by a given complex number
by a given complex number  is simply a spatial translation by a displacement of
is simply a spatial translation by a displacement of  . The multiplication operation need not be isometric (due to the presence of the dilation
. The multiplication operation need not be isometric (due to the presence of the dilation  ), but observe that both the addition and multiplication operations are conformal
), but observe that both the addition and multiplication operations are conformal
(angle-preserving) and also orientation-preserving (a counterclockwise
loop will transform to another counterclockwise loop, and similarly for
clockwise loops). As we shall see later, these conformal and
orientation-preserving properties of the addition and multiplication
maps will extend to the larger class of complex differentiable maps (at least outside of critical points of the map), and are an important aspect of the geometry of such maps.
 of a non-zero complex number
of a non-zero complex number  to lie in a fundamental domain of the
to lie in a fundamental domain of the 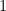 -torus
-torus 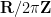 , such as the half-open interval
, such as the half-open interval 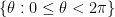 or
or  , in order to recover a unique parameterisation (at the cost of creating a branch cut at one point of the unit circle). Traditionally, the fundamental domain that is most often used is the half-open interval
, in order to recover a unique parameterisation (at the cost of creating a branch cut at one point of the unit circle). Traditionally, the fundamental domain that is most often used is the half-open interval  . The unique argument of
. The unique argument of  that lies in this interval is called the standard argument of
that lies in this interval is called the standard argument of  and is denoted
and is denoted  , and
, and  is called the standard branch of the argument function. Thus for instance
is called the standard branch of the argument function. Thus for instance 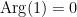 ,
, 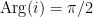 ,
,  , and
, and 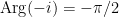 . Observe that the standard branch of the argument has a discontinuity on the negative real axis
. Observe that the standard branch of the argument has a discontinuity on the negative real axis  , which is the branch cut
, which is the branch cut
of this branch. Changing the fundamental domain used to define a branch
of the argument can move the branch cut around, but cannot eliminate it
completely, due to non-trivial monodromy
(if one continuously loops once counterclockwise around the origin, and
varies the argument continuously as one does so, the argument will
increment by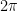 , and so no branch of the argument function can be continuous at every point on the loop).
, and so no branch of the argument function can be continuous at every point on the loop).
The multiplication formula (8) resembles the multiplication formula
for the real exponential function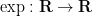 . The two formulae can be unified through the famous Euler formula involving the complex exponential
. The two formulae can be unified through the famous Euler formula involving the complex exponential  . There are many ways to define the complex exponential. Perhaps the most natural is through the ordinary differential equation
. There are many ways to define the complex exponential. Perhaps the most natural is through the ordinary differential equation  with boundary condition
with boundary condition  .
.
However, as we have not yet set up a theory of complex differentiation,
we will proceed (at least temporarily) through the device of Taylor series. Recalling that the real exponential function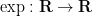 has the Taylor expansion
has the Taylor expansion

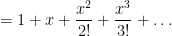 which is absolutely convergent for any real
which is absolutely convergent for any real  , one is led to define the complex exponential function
, one is led to define the complex exponential function  by the analogous expansion
by the analogous expansion
 noting from (4) that the absolute convergence of the real exponential
noting from (4) that the absolute convergence of the real exponential 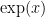 for any
for any 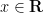 implies the absolute convergence of the complex exponential for any
implies the absolute convergence of the complex exponential for any  . We also frequently write
. We also frequently write 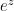 for
for  . The multiplication formula (10) for the real exponential extends to the complex exponential:
. The multiplication formula (10) for the real exponential extends to the complex exponential:
 with the familiar Taylor expansions
with the familiar Taylor expansions

 and
and

 for the (real) sine and cosine functions, one obtains Euler formula
for the (real) sine and cosine functions, one obtains Euler formula
for any real number ; in particular we have the famous identities
; in particular we have the famous identities
and
We now see that the multiplication formula (8) can be written as a special form
 of (12); similarly, de Moivre’s formula takes the simple and intuitive form
of (12); similarly, de Moivre’s formula takes the simple and intuitive form
 From (12) and (13) we also see that the exponential function basically transforms Cartesian coordinates to polar coordinates:
From (12) and (13) we also see that the exponential function basically transforms Cartesian coordinates to polar coordinates:
 Later on in the course we will study (the various branches of) the
Later on in the course we will study (the various branches of) the
logarithm function that inverts the complex exponential, thus converting
polar coordinates back to Cartesian ones.
From (13) and (1), together with the easily verified identity
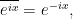 we see that we can recover the trigonometric functions
we see that we can recover the trigonometric functions  from the complex exponential by the formulae
from the complex exponential by the formulae
(Indeed, if one wished, one could take these identities as the definition
of the sine and cosine functions, giving a purely analytic way to
construct these trigonometric functions.) From these identities one can
derive all the usual trigonometric identities from the basic properties
of the exponential (and in particular (12)). For instance, using a little bit of high school algebra we can prove the familiar identity
 from (16):
from (16):
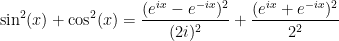
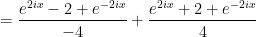
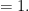 Thus, in principle at least, one no longer has a need to memorize
Thus, in principle at least, one no longer has a need to memorize
all the different trigonometric identities out there, since they can now
all be unified as consequences of just a handful of basic identities
for the complex exponential, such as (12), (14), and (15).
In view of (16), it is now natural to introduce the complex sine and cosine functions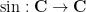 and
and  by the formula
by the formula
These complex trigonometric functions no longer
have a direct trigonometric interpretation (as one cannot easily
develop a theory of complex angles), but they still inherit almost all
of the algebraic properties of their real-variable counterparts. For
instance, one can repeat the above high school algebra computations verbatim to conclude that
for all . (We caution however that this does not imply that
. (We caution however that this does not imply that 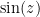 and
and  are bounded in magnitude by
are bounded in magnitude by 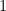 – note carefully the lack of absolute value signs outside of
– note carefully the lack of absolute value signs outside of 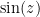 and
and  in the above formula! See also Exercise 16
in the above formula! See also Exercise 16
below.) Similarly for all of the other trigonometric identities. (Later
on in this series of lecture notes, we will develop the concept of analytic continuation, which can explain why so many real-variable algebraic identities naturally extend to their complex counterparts.) From (11)
we see that the complex sine and cosine functions have the same Taylor
series expansion as their real-variable counterparts, namely

 and
and
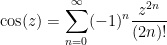
 The formulae (17) for the complex sine and cosine functions greatly resemble those of the hyperbolic trigonometric functions
The formulae (17) for the complex sine and cosine functions greatly resemble those of the hyperbolic trigonometric functions 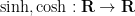 , defined by the formulae
, defined by the formulae
 Indeed, if we extend these functions to the complex domain by defining
Indeed, if we extend these functions to the complex domain by defining  to be the functions
to be the functions
 then on comparison with (17) we obtain the complex identities
then on comparison with (17) we obtain the complex identities
or equivalently
for all complex .
.
Thus we see that once we adopt the perspective of working over the
complex numbers, the hyperbolic trigonometric functions are “rotations
by 90 degrees” of the ordinary trigonometric functions; this is a simple
example of what physicists call a Wick rotation.
In particular, we see from these identities that any trigonometric
identity will have a hyperbolic counterpart, though due to the presence
of various factors of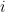 , the signs may change as one passes from trigonometric to hyperbolic functions or vice versa (a fact quantified by Osborne’s rule). For instance, by substituting (19) or (20) into (18) (and replacing
, the signs may change as one passes from trigonometric to hyperbolic functions or vice versa (a fact quantified by Osborne’s rule). For instance, by substituting (19) or (20) into (18) (and replacing  by
by  or
or 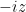 as appropriate), we end up with the analogous identity
as appropriate), we end up with the analogous identity
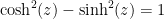 for the hyperbolic trigonometric functions. Similarly for all other
for the hyperbolic trigonometric functions. Similarly for all other
trigonometric identities. Thus we see that the complex exponential
single-handedly unites the trigonometry, hyperbolic trigonometry, and
the real exponential function into a single coherent theory!
 .
.
246A, Notes 0: the complex numbers
18 September, 2016 in 246A - complex analysis, math.CV, math.RA | Tags: complex numbers, exponentiation, trigonometry
numbers; all else is the work of man”. The truth of this statement
(literal or otherwise) is debatable; but one can certainly view the
other standard number systems
- The integers
are the additive completion of the natural numbers
(the minimal additive group that contains a copy of
).
- The rationals
are the multiplicative completion of the integers
(the minimal field that contains a copy of
).
- The reals
are the metric completion of the rationals
(the minimal complete metric space that contains a copy of
).
- The complex numbers
are the algebraic completion of the reals
(the minimal algebraically closed field that contains a copy of
).
in place of metric spaces.) The definition of the complex numbers as
the algebraic completion of the reals does not suffer from such a
non-circularity issue, but a certain amount of field theory is required
to work with this definition initially. For the purposes of quickly
constructing the complex numbers, it is thus more traditional to first
define
The two equivalent definitions of
– as the algebraic closure, and as a quadratic extension, of the reals
respectively – each reveal important features of the complex numbers in
applications. Because
is algebraically closed, all polynomials over the complex numbers split
completely, which leads to a good spectral theory for both
finite-dimensional matrices and infinite-dimensional operators; in
particular, one expects to be able to diagonalise most matrices and
operators. Applying this theory to constant coefficient ordinary
differential equations leads to a unified theory of such solutions, in
which real-variable ODE behaviour such as exponential growth or decay,
polynomial growth, and sinusoidal oscillation all become aspects of a
single object, the complex exponential
Applying this theory more generally to diagonalise arbitrary
translation-invariant operators over some locally compact abelian group,
one arrives at Fourier analysis,
which is thus most naturally phrased in terms of complex-valued
functions rather than real-valued ones. If one drops the assumption that
the underlying group is abelian, one instead discovers the
representation theory of unitary representations,
which is simpler to study than the real-valued counterpart of
orthogonal representations. For closely related reasons, the theory of
complex Lie groups is simpler than that of real Lie groups.
Meanwhile, the fact that the complex numbers are a quadratic extension
of the reals lets one view the complex numbers geometrically as a
two-dimensional plane over the reals (the Argand plane).
Whereas a point singularity in the real line disconnects that line, a
point singularity in the Argand plane leaves the rest of the plane
connected (although, importantly, the punctured plane is no longer simply connected).
As we shall see, this fact causes singularities in complex analytic
functions to be better behaved than singularities of real analytic
functions, ultimately leading to the powerful residue calculus
for computing complex integrals. Remarkably, this calculus, when
combined with the quintessentially complex-variable technique of contour shifting, can also be used to compute some (though certainly not all) definite integrals of real-valued
functions that would be much more difficult to compute by purely
real-variable methods; this is a prime example of Hadamard’s famous
dictum that “the shortest path between two truths in the real domain
passes through the complex domain”.
Another important geometric feature of the Argand plane is the angle
between two tangent vectors to a point in the plane. As it turns out,
the operation of multiplication by a complex scalar preserves the
magnitude and orientation of such angles; the same fact is true for any
non-degenerate complex analytic mapping, as can be seen by performing a
Taylor expansion to first order. This fact ties the study of complex
mappings closely to that of the conformal geometry
of the plane (and more generally, of two-dimensional surfaces and
domains). In particular, one can use complex analytic maps to
conformally transform one two-dimensional domain to another, leading
among other things to the famous Riemann mapping theorem, and to the classification of Riemann surfaces.
If one Taylor expands complex analytic maps to second order rather than
first order, one discovers a further important property of these maps,
namely that they are harmonic.
This fact makes the class of complex analytic maps extremely rigid and
well behaved analytically; indeed, the entire theory of elliptic PDE now
comes into play, giving useful properties such as elliptic regularity and the maximum principle.
In fact, due to the magic of residue calculus and contour shifting, we
already obtain these properties for maps that are merely complex
differentiable rather than complex analytic, which leads to the striking
fact that complex differentiable functions are automatically analytic
(in contrast to the real-variable case, in which real differentiable
functions can be very far from being analytic).
The geometric structure of the complex numbers (and more generally of
complex manifolds and complex varieties), when combined with the
algebraic closure of the complex numbers, leads to the beautiful subject
of complex algebraic geometry, which motivates the much more
general theory developed in modern algebraic geometry. However, we will
not develop the algebraic geometry aspects of complex analysis here.
Last, but not least, because of the good behaviour of Taylor series in
the complex plane, complex analysis is an excellent setting in which to
manipulate various generating functions, particularly Fourier series
and the complex analytic behaviour of the Dirichlet or Fourier series,
particularly with regard to its poles and other singularities. This
turns out to be a particularly handy dictionary in analytic number theory, for instance relating the distribution of the primes to the Riemann zeta function. Nowadays, many of the analytic number theory results first obtained through complex analysis (such as the prime number theorem)
can also be obtained by more “real-variable” methods; however the
complex-analytic viewpoint is still extremely valuable and illuminating.
We will frequently touch upon many of these connections to other fields
of mathematics in these lecture notes. However, these are mostly side
remarks intended to provide context, and it is certainly possible to
skip most of these tangents and focus purely on the complex analysis
material in these notes if desired.
Note: complex analysis is a very visual subject, and one should draw
plenty of pictures while learning it. I am however not planning to put
too many pictures in these notes, partly as it is somewhat inconvenient
to do so on this blog from a technical perspective, but also because
pictures that one draws on one’s own are likely to be far more useful to
you than pictures that were supplied by someone else.
— 1. The construction and algebra of the complex numbers —
Note: this section will be far more algebraic in nature than the rest ofthe course; we are concentrating almost all of the algebraic
preliminaries in this section in order to get them out of the way and
focus subsequently on the analytic aspects of the complex numbers.
Thanks to the laws of high-school algebra, we know that the real numbers
it is endowed with the arithmetic operations of addition, subtraction,
multiplication, and division, as well as the additive identity
The algebraic structure of the reals does have one drawback though – not
all (non-trivial) polynomials have roots! Most famously, the polynomial
equation
As mentioned in the introduction, one traditional way to define the complex numbers
Definition 1 (The complex numbers) A field of complex numbers is a field(We will take the existence of the real numbersthat contains the real numbers
as a subfield, as well as a root
of the equation
. (Thus, strictly speaking, a field of complex numbers is a pair
, but we will almost always abuse notation and use
as a metonym for the pair
.) Furthermore,
is generated by
and
, in the sense that there is no subfield of
, other than
itself, that contains both
and
; thus, in the language of field extensions, we have
.
as a given in this course; constructions of the real number system can
of course be found in many real analysis texts, including my own.)
Definition 1 is short, but proposing it as a definition of the complex numbers raises some immediate questions:
- (Existence) Does such a field
even exist?
- (Uniqueness) Is such a field
unique (up to isomorphism)?
- (Non-arbitrariness) Why the square root of
? Why not adjoin instead, say, a fourth root of
, or the solution to some other algebraic equation? Also, could one iterate the process, extending
further by adding more roots of equations?
We begin with existence. One can construct the complex numbers quite
explicitly and quickly using the Argand plane construction; see Remark 7
below. However, from the perspective of higher mathematics, it is more
natural to view the construction of the complex numbers as a special
case of the more general algebraic construction that can extend any
field
Starting with the real numbers
as a real variable; but if one were to generalise this construction to
positive characteristic fields, and particularly finite fields, then one
can run into difficulties if polynomials are not treated formally, due
to the fact that two distinct formal polynomials might agree on all
inputs in a given finite field (e.g. the polynomials
of polynomials has a pretty good algebraic structure, in particular the
usual operations of addition, subtraction, and multiplication on
polynomials, together with the zero polynomial
If a unital commutative ring fails to be field, then it will instead possess a number of non-trivial ideals. The only ideal we will need to consider here is the principal ideal
We now define
If we define
The only remaining thing to verify is that
Because the polynomial
Since
Remark 2 One can think of the action of passing from a ringto a quotient
by some ideal
as the action of forcing some relations to hold between the various elements of
, by requiring all the elements of the ideal
(or equivalently, all the generators of
) to vanish. Thus one can think of
as the ring formed by adjoining a new element
to the existing ring
and then demanding the constraint
.
With this perspective, the main issues to check in order to obtain a
complex field are firstly that these relations do not collapse the ring
so much that two previously distinct elements of
become equal, and secondly that all the non-zero elements become
invertible once the relations are imposed, so that we obtain a field
rather than merely a ring or integral domain.
Remark 3 It is instructive to compare the complex fieldUniqueness of, formed by adjoining the square root of
to the reals, with other commutative rings such as the dual numbers
(which adjoins an additional square root of
to the reals) or the split complex numbers
(which adjoins a new root of
to the reals). The latter two objects are perfectly good rings, but are
not fields (they contain zero divisors, and the first ring even
contains a nilpotent). This is ultimately due to the reducible nature of
the polynomialsand
in
.
Exercise 4 (Uniqueness up to isomorphism) Suppose that one has two complex fieldsNow that we have existence and uniqueness up to isomorphism, it is safe to designate one of the complex fieldsand
. Show that there is a unique field isomorphism
that maps
to
and is the identity on
.
complex field; the other complex fields out there will no longer be of
much importance in this course (or indeed, in most of mathematics), with
one small exception that we will get to later in this section. One can,
if one wishes, use the above abstract algebraic construction
is not terribly relevant for the purposes of actually doing complex
analysis, much as the question of whether to construct the real numbers
using Dedekind cuts, equivalence classes of Cauchy sequences, or some
other construction is not terribly relevant for the purposes of actually
doing real analysis. So, from here on out, we will no longer refer to
the precise construction of
Exercise 5 Letbe an arbitrary field, let
be the ring of polynomials with coefficients in
, and let
be an irreducible polynomial in
of degree at least two. Show that
is a field containing an embedded copy of
, as well as a root
of the equation
, and that this field is generated by
and
. Also show that all such fields are unique up to isomorphism. (This field
is an example of a field extension of
,
the further study of which can be found in any advanced undergraduate
or early graduate text on algebra, and is the starting point in
particular for the beautiful topic of Galois theory, which we will not discuss here.)
Exercise 6 Letbe an arbitrary field. Show that every non-constant polynomial
in
can be factored as the product
of irreducible non-constant polynomials. Furthermore show that this factorisation is unique up to permutation of the factors
,
and multiplication of each of the factors by a constant (with the
product of all such constants being one). In other words: the
polynomiail ringis a unique factorisation domain.
Remark 7 (Real and imaginary coordinates) As a complex fieldis spanned (over
) by the linearly independent elements
and
, we can write
with each elementof
having a unique representation of the form
, thus
for real. The addition, subtraction, and multiplication operations can then be written down explicitly in these coordinates as
and with a bit more work one can compute the division operation as
if. One could take these coordinate representations as the definition of the complex field
and its basic arithmetic operations, and this is indeed done in many
texts introducing the complex numbers. In particular, one could take the
Argand planeas the choice of complex field, where we identify each point
in
with
(so for instance
becomes endowed with the multiplication operation
).
This is a very concrete and direct way to construct the complex
numbers; the main drawback is that it is not immediately obvious that
the field axioms are all satisfied. For instance, the associativity of
multiplication is rather tedious to verify in the coordinates of the
Argand plane. In contrast, the more abstract algebraic construction of
the complex numbers given above makes it more evident what the source of
the field structure onis, namely the irreducibility of the polynomial
.
Remark 8 Because of the Argand plane construction, we will sometimes refer to the spaceElements ofof complex numbers as the complex plane. We should warn, though, that in some areas of mathematics, particularly in algebraic geometry,
is viewed as a one-dimensional complex vector space (or a one-dimensional complex manifold or complex variety), and so
is sometimes referred to in those cases as a complex line. (Similarly, Riemann surfaces, which from a real point of view are two-dimensional surfaces, can sometimes be referred to as complex curves in the literature; the modular curve
is a famous instance of this.) In this current course, though, the
topological notion of dimension turns out to be more important than the
algebraic notions of dimension, and as such we shall generally refer toas a plane rather than a line.
the terminology is colourful, but despite the name, imaginary numbers
have precisely the same first-class mathematical object status as real
numbers. If
Remark 9 We noted earlier that the equationAs with any other field, we can raise a complex numberhad no solutions in the reals because
was always positive. In other words, the properties of the order relation
on
prevented the existence of a root for the equation
. As
does have a root for
, this means that the complex numbers
cannot be ordered in the same way that the reals are ordered (that is
to say, being totally ordered, with the positive numbers closed under
both addition and multiplication). Indeed, one usually refrains from
putting any order structure on the complex numbers, so that statements
such asfor complex numbers
are left undefined (unless
are real, in which case one can of course use the real ordering). In particular, the complex number
is considered to be neither positive nor negative, and an assertion such as
is understood to implicitly carry with it the claim that
are real numbers and not just complex numbers. (Of course, if one
really wants to, one can find some total orderings to place on, e.g. lexicographical ordering on the real and imaginary parts. However, such orderings do not interact too well with the algebraic structure of
and are rarely useful in practice.)
other than an integer; we will return to such exponentiation operations
later in the course, though we will at least define the complex
exponential
By definition, a complex field
is real. Geometrically, complex conjugation is the operation of
reflection in the complex plane across the real axis. It is clearly an involution in the sense that it is its own inverse:
Remark 10 Any field automorphism ofObserve that if we multiply a complex numberhas to map
to a root of
, and so the only field automorphisms of
that preserve the real line are the identity map and the conjugation map; conversely, the real line is the subfield of
fixed by both of these automorphisms. In the language of Galois theory, this means that
is a Galois extension of
, with Galois group
consisting of two elements. There is a certain sense in which one can think of the complex numbers (or more precisely, the scheme
of complex numbers) as a double cover of the real numbers (or more precisely, the scheme
of real numbers), analogous to how the boundary of a Möbius strip can
be viewed as a double cover of the unit circle formed by shrinking the
width of the strip to zero. (In this analogy, points on the unit circle
correspond to specific models of the real number system, and lying above each such point are two specific models
,
of the complex number system; this analogy can be made precise using
Grothendieck’s “functor of points” interpretation of schemes.) The
operation of complex conjugation is then analogous to the operation of monodromy
caused by looping once around the base unit circle, causing the two
complex fields sitting above a real field to swap places with each
other. (This analogy is not quite perfect, by the way, because the
boundary of a Möbius strip is not simply connected and can in turn be
finitely covered by other curves, whereas the complex numbers are
algebraically complete and admit no further finite extensions; one
should really replace the unit circle here by something with a
two-element fundamental group, such as the projective planethat is double covered by the sphere
, but this is harder to visualize.) The analogy between (absolute)
Galois groups and fundamental groups suggested by this picture can be
made precise in scheme theory by introducing the concept of an étale fundamental group, which unifies the two concepts, but developing this further is well beyond the scope of this course; see this book of Szamuely for further discussion.
for any non-zero complex number
— 2. The geometry of the complex numbers —
The norm form on the reals, which as remarked previously is no longer present
directly in the complex numbers. (One can also view the positive
definiteness of the norm form as a consequence of the topological
connectedness of the punctured complex plane
One consequence of positive definiteness is that the bilinear form
(which implies the usual permutations of this inequality, such as
(and hence also
The norm
thus for instance
As with any other normed vector space, the norm
which in turn generates all the usual topological notions such as the
concept of an open set, closed set, compact set, connected set, and
boundary of a set; the notion of a limit of a sequence
or equivalently if the inverse image of any open set is open. Again,
using the Argand plane representation, these notions coincide with their
counterparts on the Euclidean plane
As usual, if a sequence
for the reciprocal of a complex number, we also see that division is a
continuous operation as long as the denominator is non-zero, thus
From (7) we see that
As with the reals, we have the fundamental fact that any formal series
We will occasionally have need to deal with series that are only
conditionally convergent rather than absolutely convergent, but in most
of our applications the only series we will actually evaluate are the
absolutely convergent ones. Many of the limit laws imply analogues for
series, thus for instance
(or even just convergent). We will not write down an exhaustive list of
such series laws here.
An important role in complex analysis is played by the unit circle
and so this indeed has the geometric structure of a unit circle.
Elements of the unit circle will be referred to in these notes as phases. Every non-zero complex number
From (4) we see that the unit circle
respectively. Thus polar coordinates are very convenient for performing
complex multiplication, although they turn out to be atrocious for
performing complex addition. (This can be contrasted with the usual
Cartesian coordinates
which are very convenient for performing complex addition and mildly
inconvenient for performing complex multiplication.) In the language of
group theory, the polar decomposition splits the multiplicative complex
group
If
must either be a rotation around the origin, or a reflection around a
line. The former operation is orientation preserving, and the latter is
orientation reversing. Since the map
taking real and imaginary parts, we recover the familiar trigonometric addition formulae
Exercise 11Every non-zero complex number
- (i) Let
be an isometry of the Euclidean plane that fixes the origin
. Show that
is either a rotation around the origin by some angle
, or the reflection around some line through the origin. (Hint: try to compose
with rotations or reflections to achieve some normalisation of
, e.g. that
fixes
. Then consider what
must do to other points in the plane, such as
. Alternatively, one can use various formulae relating distances to angle, such as the sine rule or cosine rule, or the formula
for the inner product.) For this question, you may use any result you already know from Euclidean geometry or trigonometry.
- (ii) Show that all isometries
of the complex numbers take the form
or
for some complex numberand phase
.
with
and can be interpreted as an angle of counterclockwise rotation needed
to rotate the positive real axis to a position that contains
The operation
As complex multiplication is commutative and associative, it does not
matter in which order one performs the dilation and rotation operations.
Similarly, using Cartesian coordinates, we see that the operation
(angle-preserving) and also orientation-preserving (a counterclockwise
loop will transform to another counterclockwise loop, and similarly for
clockwise loops). As we shall see later, these conformal and
orientation-preserving properties of the addition and multiplication
maps will extend to the larger class of complex differentiable maps (at least outside of critical points of the map), and are an important aspect of the geometry of such maps.
Remark 12 One can also interpret the operationsWhen performing computations, it is convenient to restrict the argument
of complex arithmetic geometrically on the Argand plane as follows. As
the addition law oncoincides with the vector addition law on
,
addition and subtraction of complex numbers is given by the usual
parallelogram rule for vector addition; thus, to add a complex numberto another
, we can translate the complex plane until the origin
gets mapped to
, and then
gets mapped to
; conversely, subtraction by
corresponds to translating
back to
. Similarly, to multiply a complex number
with another
, we can dilate and rotate the complex plane around the origin until
gets mapped to
, and then
will be mapped to
; conversely, division by
corresponds to dilating and rotating
back to
.
of this branch. Changing the fundamental domain used to define a branch
of the argument can move the branch cut around, but cannot eliminate it
completely, due to non-trivial monodromy
(if one continuously loops once counterclockwise around the origin, and
varies the argument continuously as one does so, the argument will
increment by
The multiplication formula (8) resembles the multiplication formula
for the real exponential function
However, as we have not yet set up a theory of complex differentiation,
we will proceed (at least temporarily) through the device of Taylor series. Recalling that the real exponential function
Exercise 13 Use the binomial theorem and Fubini’s theorem for (complex) doubly infinite series to conclude thatIf one compares the Taylor series for
for any complex numbers.
for any real number
and
We now see that the multiplication formula (8) can be written as a special form
logarithm function that inverts the complex exponential, thus converting
polar coordinates back to Cartesian ones.
From (13) and (1), together with the easily verified identity
(Indeed, if one wished, one could take these identities as the definition
of the sine and cosine functions, giving a purely analytic way to
construct these trigonometric functions.) From these identities one can
derive all the usual trigonometric identities from the basic properties
of the exponential (and in particular (12)). For instance, using a little bit of high school algebra we can prove the familiar identity
all the different trigonometric identities out there, since they can now
all be unified as consequences of just a handful of basic identities
for the complex exponential, such as (12), (14), and (15).
In view of (16), it is now natural to introduce the complex sine and cosine functions
These complex trigonometric functions no longer
have a direct trigonometric interpretation (as one cannot easily
develop a theory of complex angles), but they still inherit almost all
of the algebraic properties of their real-variable counterparts. For
instance, one can repeat the above high school algebra computations verbatim to conclude that
for all
below.) Similarly for all of the other trigonometric identities. (Later
on in this series of lecture notes, we will develop the concept of analytic continuation, which can explain why so many real-variable algebraic identities naturally extend to their complex counterparts.) From (11)
we see that the complex sine and cosine functions have the same Taylor
series expansion as their real-variable counterparts, namely
or equivalently
for all complex
Thus we see that once we adopt the perspective of working over the
complex numbers, the hyperbolic trigonometric functions are “rotations
by 90 degrees” of the ordinary trigonometric functions; this is a simple
example of what physicists call a Wick rotation.
In particular, we see from these identities that any trigonometric
identity will have a hyperbolic counterpart, though due to the presence
of various factors of
trigonometric identities. Thus we see that the complex exponential
single-handedly unites the trigonometry, hyperbolic trigonometry, and
the real exponential function into a single coherent theory!
Exercise 14The next exercise gives a special case of the fundamental theorem of algebra, when considering the roots of polynomials of the specific form
- (i) If
is a positive integer, show that the only complex number solutions to the equation
are given by the
complex numbers
for
; these numbers are thus known as the
roots of unity. Conclude the identity
for any complex number
.
- (ii) Show that the only compact subgroups
of the multiplicative complex numbers
are the unit circle
and the
roots of unity
for. (Hint: there are two cases, depending on whether
is a limit point of
or not.)
- (iii) Give an example of a non-compact subgroup of
.
- (iv) (Warning: this one is tricky.) Show that the only connected closed subgroups of
are the whole group
, the trivial group
, and the one-parameter groups of the form
for some non-zero complex number
.
Exercise 15 Show that ifis a non-zero complex number and
is a positive integer, then there are exactly
distinct solutions to the equation
, and any two such solutions differ (multiplicatively) by an
root of unity. In particular, a non-zero complex number
has two square roots, each of which is the negative of the other. What happens when
?
Exercise 16 Letbe a sequence of complex numbers. Show that
is bounded if and only if the imaginary part of
is bounded, and similarly with
replaced by
.
Exercise 17 (This question was drawn from a previous version of this course taught by Rowan Killip.) Letbe distinct complex numbers, and let
be a positive real that is not equal to
.
- (i) Show that the set
defines a circle in the complex plane. (Ideally, you should be able to
do this without breaking everything up into real and imaginary parts.)- (ii) Conversely, show that every circle in the complex plane arises in such a fashion (for suitable choices of
, of course).
- (iii) What happens if
?
- (iv) Let
be a circle that does not pass through the origin. Show that the image of
under the inversion map
is a circle. What happens if
is a line? What happens if the
passes through the origin (and one then deletes the origin from
before applying the inversion map)?
Exercise 18 Ifis a complex number, show that
.
Εγγραφή σε:
Σχόλια (Atom)

